Lab: Ballistic Pendulum
May Soe Moe
Lab Partners: Day 1: Steven Castro, Stephanie Flores, Henry Wang, Tomas Pacual, Garrett Giordano, Jesus Gonzalez, Ben Chen
Day 2: Steven Castro, Stephanie Flores, Henry Wang, Ben Chen
Date: 26-April-2017, 3-May-2017
Objective: To determine the firing speed of a ball from a spring-loaded gun and to determine how far the ball lands after it is launched horizontally
Introduction: There are two parts for this lab, which we performed on two separate days. For the first part, using a spring-loaded gun, we will fire a ball into a nylon block, supported with four vertical springs. The ball will be stuck into the block, and the block, together with the ball, will rise up to some angle, which we will measure with the angle indicator on the apparatus. To figure out the ball's firing speed, we will use conservation of momentum (momentum p=mv) to write an expression representing the initial speed of the ball, immediately after the collision. Conservation of momentum states that momentum is conserved if there is no net external forces acting on a system of objects, and those objects will have equal and opposite force acting on each other. For the second part, we lay out the apparatus above a pile of books. We estimate where the ball would land after the launch horizontally, and test it out. We put the carbon paper on the floor approximately at the point where it would land to mark out its final position. We will take appropriate measurements such as the height of the spring loaded gun from the ground, and the distance of the final position of the ball from the apparatus. We will use conservation of energy to get the initial firing speed of the ball and compare the two velocities we got from part 1 and part 2 of this lab.
Experimental Procedure:
Part 1:
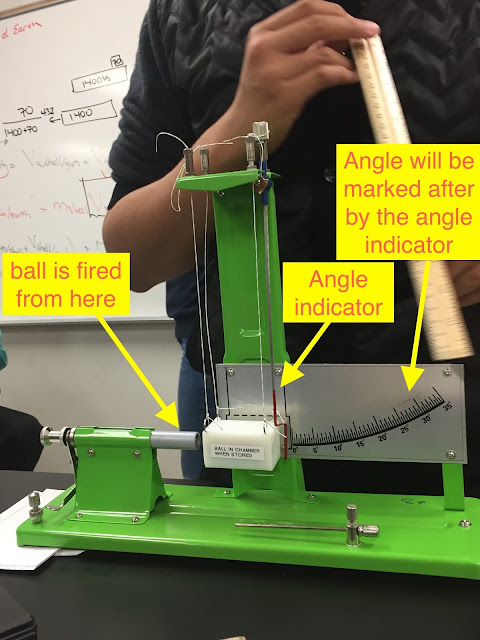
(1) Our apparatus looks like this:
May Soe Moe
Lab Partners: Day 1: Steven Castro, Stephanie Flores, Henry Wang, Tomas Pacual, Garrett Giordano, Jesus Gonzalez, Ben Chen
Day 2: Steven Castro, Stephanie Flores, Henry Wang, Ben Chen
Date: 26-April-2017, 3-May-2017
Objective: To determine the firing speed of a ball from a spring-loaded gun and to determine how far the ball lands after it is launched horizontally
Introduction: There are two parts for this lab, which we performed on two separate days. For the first part, using a spring-loaded gun, we will fire a ball into a nylon block, supported with four vertical springs. The ball will be stuck into the block, and the block, together with the ball, will rise up to some angle, which we will measure with the angle indicator on the apparatus. To figure out the ball's firing speed, we will use conservation of momentum (momentum p=mv) to write an expression representing the initial speed of the ball, immediately after the collision. Conservation of momentum states that momentum is conserved if there is no net external forces acting on a system of objects, and those objects will have equal and opposite force acting on each other. For the second part, we lay out the apparatus above a pile of books. We estimate where the ball would land after the launch horizontally, and test it out. We put the carbon paper on the floor approximately at the point where it would land to mark out its final position. We will take appropriate measurements such as the height of the spring loaded gun from the ground, and the distance of the final position of the ball from the apparatus. We will use conservation of energy to get the initial firing speed of the ball and compare the two velocities we got from part 1 and part 2 of this lab.
Experimental Procedure:
Part 1:
(1) Our apparatus looks like this:
(2) The ball is fired from the spring-loaded gun and it went into the nylon block, supported with four vertical strings.
(3) There is a hole inside the nylon block, so that the ball will stay inside the block after the collision.
(4) After the collision, the ball along with the block moved to some maximum vertical angle, that is indicated by an angle indicator from our apparatus.
(5) The collision also caused change in height of the block.
(6) We repeated the process about six times to get an average angle.
Part 1 Calculations:
 |
| Firing speed of the ball resulted as 4.9m/s. |
(7) Our calculations give us the firing speed of the ball to be 4.9 m/s.
Part 2:
Experimental Procedure:
(1) We set up our apparatus as below:
 |
| Our set up for Part 2 of the Ballistic Pendulum lab |
(2) The height of the pile of the books to the spring loaded gun was measured as 20.5 cm (0.205m).
(3) We estimated where the ball would land after firing it and put a blank sheet of white paper and carbon paper on top. We repeated this process for four times.
(4) After that we removed the carbon paper and measured the distance of the ball's position after it landed from the apparatus, using a meter stick.
 |
| Measuring the distance from the apparatus to where the ball landed |
Part 2 Calculations:
 |
| Firing speed of the ball came out to be 5.175 m/s |
Conclusions:
The first part's initial firing speed is 4.9 m/s and the second part's initial firing speed is 5.175 m/s. There are uncertainties in our data that caused the difference in two firing speed of the ball. Our measurements of the angles, masses of the block and the ball, the height of the apparatus, height of the book pile, and the distances we measured away from the apparatus to where the ball landed were not exact numbers as we calculated. We used the average of he angles and distances we got from our trials to calculate it. The other factor to consider about the difference in firing speed is that we did this lab in two separate parts and two separate days. It is possible that we used different apparatus for two parts. But the difference between two results of the firing speed from the calculation was not large.